Winter school in singularities and low-dimensional topology

The Erdős Center semester on "Singularities and low-dimensional topology" will kick off with a 2-phase winter school to introduce early stage researchers (MSc students, PhD students, postdocs) to some key concepts in singularities and low-dimensional topology.

Download the programme of the Winter School and the schedule of the lightning talks here.

Here are some practical information about getting to and commuting in Budapest.

Please fill out this questionnaire on the Winter School.

Notes and video recordings

Phase 1 (pre-school)
- Vera Vértesi: Notes, Lecture 1, Lecture 2
- Marco Marengon: Notes, Lecture 1, Lecture 2
- András Stipsicz: Lecture 1, Lecture 2
- András Némethi: Lecture 1, Lecture 2

Phase 2 (school)
- Kyle Hayden: Notes available on Kyle Hayden's website, Lecture 1, Lecture 2, Lecture 3, Lecture 4
- András Némethi: Lecture 1, Lecture 2, Lecture 3, Lecture 4
- Duco van Straten: Lecture 1, Lecture 2, Lecture 3, Lecture 4
- Michael Wong: Lecture 1, Lecture 2, Lecture 3, Lecture 4

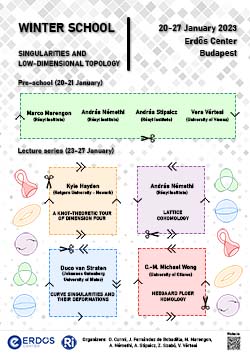
Phase 1 (pre-school)

Phase 1 is primarily aimed at motivated MSc students and early-stage PhD students, and will consist of a 2-day intense topological preparation for phase 2.

Dates: 20-21 January 2023
Location: Rényi Institute
Pre-requisites: Manifolds, vector bundles, homology, cohomology, Chern classes.
Topics include: Morse homology, Heegaard splittings, Kirby calculus, plumbed 4-manifolds, resolutions of isolated singularities in low dimension.
Lecturers: Marco Marengon (Rényi Institute), András Némethi (Rényi Institute), András Stipsicz (Rényi Institute), Vera Vértesi (University of Vienna).

Phase 2 (school)

Phase 2 is aimed at the attendees of phase 1 as well as PhD students and early-stage postdocs. It will consist of 4 parallel topics courses.

Dates: 23-27 January 2023
Location: Rényi Institute
Pre-requisites: Morse homology, Heegaard splittings, Kirby calculus, plumbed 4-manifolds, resolutions of isolated singularities in low dimension.

Courses and lecturers:
1. A knot-theoretic tour of dimension four, by Kyle Hayden (Rutgers University - Newark)
    TA: Clayton McDonald (Rényi Institute)
2. Lattice cohomology, by András Némethi (Rényi Institute)
    TAs: Alexander Kubasch (Rényi Institute) and Gergő Schefler (Rényi Institute)
3. Curve singularities and their deformations, by Duco van Straten (Johannes Gutenberg University of Mainz)
    TA: Roberto Giménez Conejero (Rényi Institute)
4. Heegaard Floer homology, by C.-M. Michael Wong (University of Ottawa)
    TA: Tristan Wells (Michigan State University)

The lectures will end at noon on Friday 27 January. However, there will be an optional TA session and optional lightning talks on Friday afternoon, which you are encourage to attend if you leave Budapest late on Friday or on Saturday.